Celestial Sphere
The celestial sphere is a modern take on the {\emph Geocentric Model} of the Universe that gives us a model of the sky on which we can map angular coordinates. The geocentric model placed the Earth at the center (geo-centric) of the Universe and placed a series of concentric spheres of varying radius around the Earth. In at least one description, these perfectly spherical shells were thought to carry the Moon, Mercury, Venus, the Sun, Mars, Jupiter, and Saturn, and then just all of the stars in successively larger-radii shells. At this time, there was no concept of forces – much less forces acting at a distance like we know gravity to do today. The geocentric model worked. With modifications and slight rearranging, astronomers of antiquity were able to tabulate and {\emph predict} the locations of celestial bodies to precision enough to convince collaborators that the geocentric model was working. Only increasingly apparent inaccuracies brought on by the advent of the telescope and the eventual `inelegance' of the geocentric model, did the heliocentric model ever prevail.
While human cultures of antiquity might have considered the Universe as a series of concentric shells ultimately centered on the Earth, today we know that the Sun is located at the center of our Solar System and that the Universe itself has no defined center. Despite the change in understanding of the fundamental structure of the Universe, the notion that the Sun, Moon, and the planets have motion distinct from the background stars still loomed large. This was captured nicely in the geocentric model by {\emph all the stars} enjoying the outermost sphere to themselves.
Today we know that the motion of the Sun, the Moon, and the planets is apparent to us day by day because those objects are closer to us (as the observer). By contrast, the day-to-day motion of the stars is not apparent to us because they are so ridiculously far away from us. This idea that the stars are so fantastically distant from the Earth enables us to treat the stars as functionally static impressions on the sky day-to-day and generally month-to-month or even year-to-year. Exceptions to this apparent-static-ness can therefore only due to the high-speed motion of nearby stars.
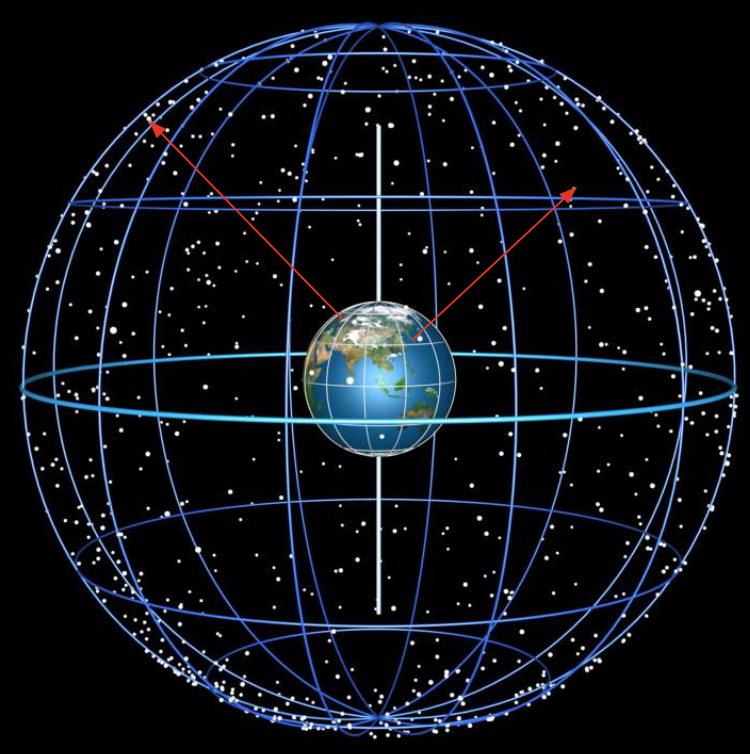
Born in antiquity, but still useful to us today is the {\bf celestial sphere}. The {\bf celestial sphere} that distant stars are so ludicrously distant from observers on Earth that they may as well be embedded in an imaginary sphere of undefined radius that surrounds the Earth – and the entire Solar System for that matter.
The {\bf Local Horizon Coordinate System (LHCS)} (a.k.a., Horizontal Coordinate System) is a spherical coordinate system defined by the observer's {\bf local horizon}. In the LHCS, the {\bf local horizon} is used as the fundamental plane to define two angles: {\bf altitude (ALT)} and {\bf azimuth (AZ)}. Ignoring any radial component, any object embedded in the {\bf celestial sphere} can be located at any instant with these two angles.
In the LHCS, zenith is defined to be directly overhead, nadir is directly underfoot, and the local horizon is the plane perpendicular to this line. This local horizon is the fundamental plane in this coordinate system, so altitude is measured from this plane, starting at $ALT = 0^\circ$ and bound by $ALT\in[0,90]^\circ$ ($90^\circ$ at zenith). The azimuth is measured eastward along the local horizon starting at North, which is defined to have $AZ=0^\circ$. The azimuth is bound by $ALT\in[0,360)\circ$. Altitudes greater than $90^\circ$ are nonsense – simply take the supplementary angle as the $ALT$ and add $180^\circ$ to the $AZ$. E.g., $(ALT, AZ) = (120^\circ,0^\circ) \rightarrow (60^\circ,180^\circ)$.
Another feature of the LCHS is the {\bf Local Meridian Plane}. The {\bf Local Meridian Plane} is the plane that contains zenith, nadir, due North, and due South. The projection of the {\bf Local Meridian Plane} onto the {\bf celestial sphere} is just the {\bf local meridian} and appears as half of a great circle connecting due North with due South and runs directly overhead. Due to the rotation of the Earth, when celestial objects cross the {\bf local meridian} the object is said to be {\bf transiting}.
The LCHS is `local'. This means it is subjected to the local geographical coordinates of the observer. Consider the celestial objects located at zenith for two different observers: one in South Korea and one in Sweden. While both observers are in the northern hemisphere, when looking directly overhead, they see very different skies. So a reported $(ALT, AZ)$ is next to meaningless if passed between the two. Further – since the Earth rotates – an object at Zenith will not remain at Zenith \todo{There are two exceptions to this statement, what are they?}. Even further – since th Earth orbits the Sun – an object that is visible during the night one day may not be visible during another night. This subjectivity of the LCHS makes it only useful for discussing objects to observers at the same time and the same place on the planet.
So how can we formulate a coordinate system utilizing the Celestial Sphere that is not so subjective to time and space?
Everything Moves
First of all, everything moves. No devised system will be perfect forever.
That said, if the objects are sufficiently far away, the map to the Celestial Sphere is good for months and even years.
Such a mapping constitutes an Astronomical Catalog.
An ephemeris is a tabulation of positions and dynamical quantities of an object or sets of objects calculated (or observed) relative to a specific time. This specific time is called an epoch. We currently use the J2000.0 epoch.
The catalog based on the J2000.0 epoch is combining all observed locations and motions in the past 50 years and rewinding/fast-forwarding them to what they'd be on January 1, 2000 at noon GMT.
Astrometric surveys are frequently undertaken to refine these positions and motions.
Due to parallax, sufficiently distant (from above) means “so distant that we can't discern the motion”.
Equatorial Coordinate System
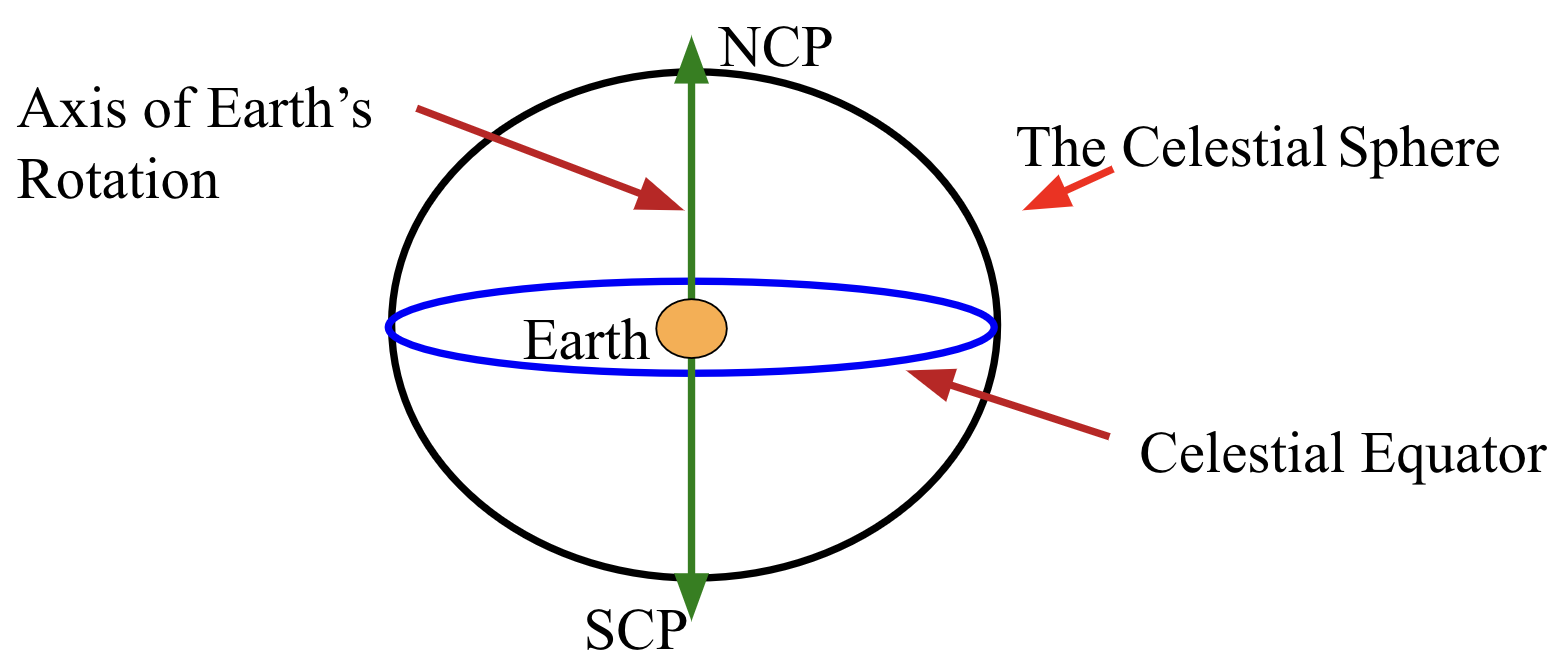
We therefore invent the Equatorial Coordinate System that uses the Earth's Equator as the fundamental plane. In this system, the Earth's Equator and rotation axis are projected out onto the Celestial Sphere. Since these don't move appreciably over months or even years, this system is fairly stable/static.
Declination
Since the fundamental plane is the plane of the Earth's Equator (called the Celestial Equator when projected onto the Celestial Sphere), we can re-use the familiar latitude lines to develop a new set of angular coordinates that are more objective than the Altitude and Azimuth we used before. We call these projected latitude lines lines of constant Declination or DEC. Declination is measured from the Celestial Equator, positive north, negative south.
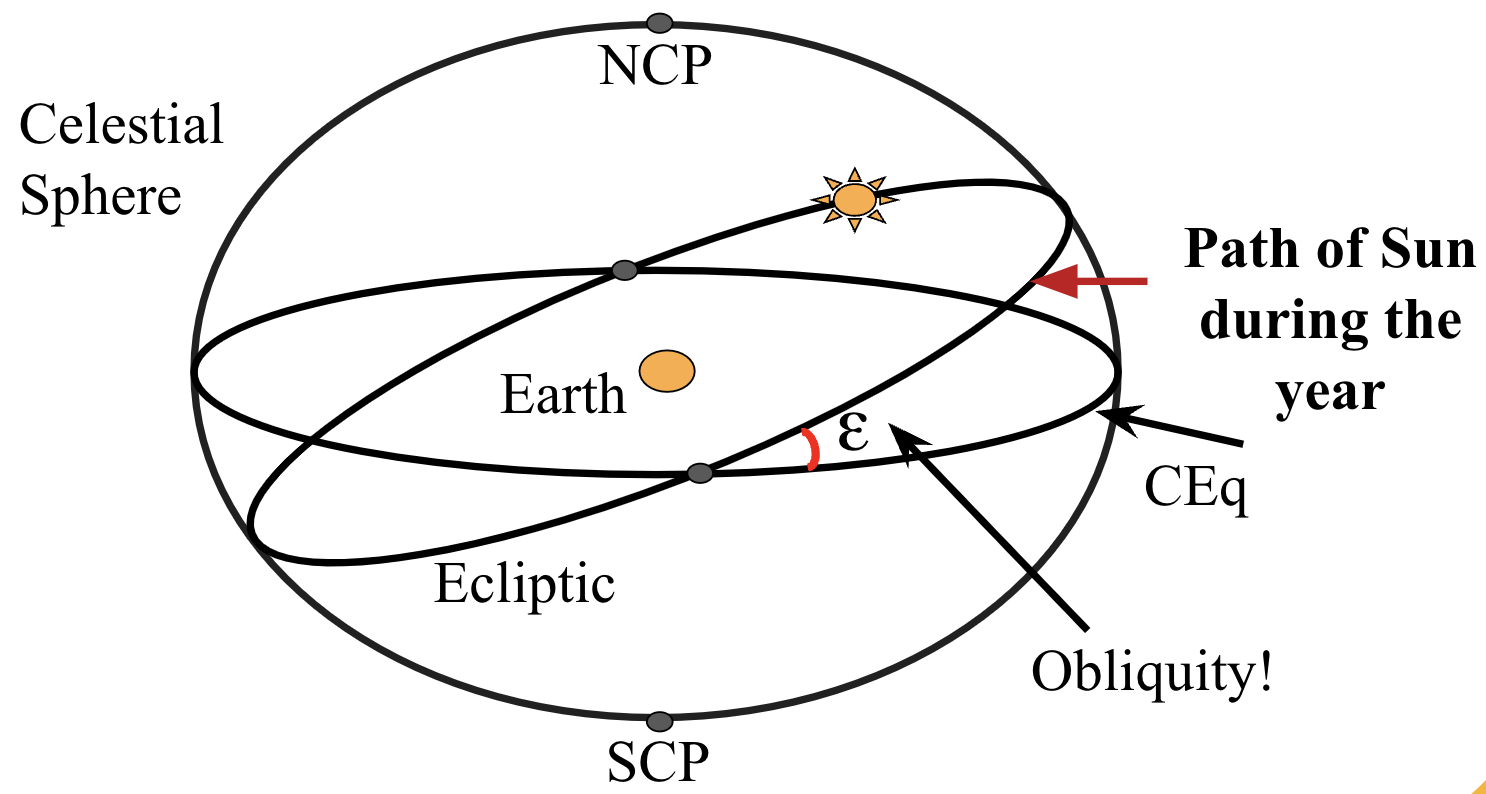
Right Ascension and Equinoxes
On Earth, we use the Prime Meridian to denote the zero-point for lines of longitude. On the Celestial Sphere under the Equatorial Coordinate System, we choose the one of the two intersections of the Celestial Equator with another plane – the Ecliptic Plane. The Ecliptic Plane is the plane of the Solar System. It's roughly perpendicular to the rotation axis of the Sun and it's therefore the plane along which the large masses in our Solar System orbit the Sun (this is due to the formation of the Solar System and fairly complex interactions between Angular Momentum and frequent collisions 4-5 Billion Years ago!). ANYWAY. The Ecliptic is the apparent path of the Sun, Moon, and the rest of the planets in our sky (our Celestial Sphere). It's a plane, so it intersects the hollow Celestial Sphere and traces a Great Circle (since the Earth is also on that plane) around the Celestial Sphere. The Plane of the Celestial Equator (also containing the Earth) constitutes another Great Circle. These two planes intersect and form a line that contains the Earth and intersects the Celestial Sphere at two points.
When the Earth-Sun line falls along these points, the rotation axis of the Earth is perpendicular to the Earth-Sun line. This is exactly the geometry of an Equinox. Said differently, these points on the Celestial Sphere are the points where the Ecliptic and Celestial Equator Great Circles intersect. The Sun crosses the Celestial Equator on the Equinox. When the Sun crosses S–>N, it's the March Equinox. When the Sun crosses N–>S, it's the September Equinox. Draw this out, think about it, ponder the orbs, make this geometry make sense to you! Let's talk about it!
The March Equinox is chosen to be the zero-point for the Equatorial Coordinate analogous to latitude – the Right Ascension or RA. RA($\alpha$) is measured eastward along the Celestial Equator starting at the March Equinox.
Milankovitch Cycles
The specific location of the CNP/CSP/CEq do change over time in cycles known as Milankovitch Cycles. These cycles are symptomatic of the Precession/Nutation of the Earth's rotation axes, Orbital Eccentricity, and Orbital Obliquity. They're due to the gravitational effects of the masses in our Solar System being more in-line with the Ecliptic than our Celestial Equator! How fun!?
Note on Units
Degrees, Minutes, Seconds – of arc!
As we're all aware, the circle is split into 360 friendly units called degrees. By the time more precise units were called for (~13th Century CE), mathematicians hadn't yet introduced the radian (1720s CE). So the units of increased precision were introduced as the minute pieces of the degree (minutes of arc) and the second-most minute pieces of the degree (secundus minutae, seconds of arc). The degree is the same one you're familiar with, denoted by the raised circle ($^\circ$). The minutes and seconds of arc (also called arcminutes and arcseconds) are denoted by one or two 'ticks', respectively. In this way, $1^\circ = 60'$ and $1' = 60''$ AND $1^\circ = 3600''$.


This history of the degree and it's close relationship to the distance the Sun appears to travel through the sky in a day-night cycle is fascinating from an anthropological lens. Enjoy!
Declination is measured in degrees, minutes of arc and seconds of arc with $360^\circ$ if traversing any Great Circle containing the CNP and CSP. Right Ascension is measured in hours, minutes, and seconds with $24^h$ if traversing the full Celestial Equator. As we shall see, changes in $\alpha$ correspond to different angular rotations of the Earth – or different times of day! These units can be confusing. Minutes of arc (or arcminutes) are NOT the same as minutes of RA. Furthermore, the notation of $\alpha$ & $\delta$ (RA & DEC) can be confusing. They can be given in their fully qualified notation $(D_1^\circ~D_2'~D_3'', R_1^h, R_2^m, R_3^s)$, as degree-decimals, or some fun mixture of the two! When looking at a very small Field of View, angular measures in $\alpha$ will frequently just be in terms of arcminutes or arcseconds. Pay careful attention to these notations and values. Be sure you understand the differences between them!
Reconciling LCHS and Eq Systems
With this Equatorial Coordinate System, an observer
For an observer at a positive latitude, $\lambda$, r ($\lambda=~39^\circ$ for MD):
- the CNP will be located at $(ALT=\lambda^\circ, AZ=0^\circ)$
- the CSP will never be above the local horizon (Why?)
- the CEq will intersect the local meridian at $(ALT=90^\circ - \lambda, AZ=180^\circ)$
- Zenith will see changing RA, depending on the local time, but the $\delta$ will always be $\lambda^\circ$
Stellarium Exercises
Using Stellarium, let’s all do the following together …
- Set location to Baltimore. Note the (lat, lon)
- Identify the Ecliptic
- Set the date to your birthday and tell me your “Sun sign” – ugh
- Explore Local Horizon Grid
- Find the Pole Star and find its (alt, az) – what should it be?
- Enable the Local Meridian
- Play time forward and watch star paths
- Explore Equatorial Grid
- Enable CEq
- Play time forward and watch star paths
- Set location to North Pole, South Pole, Equator, etc
- Determine (alt, az) of a star in Orion, determine (RA, DEC)
- Determine exact time (ET) of March Equinox
- Back in Baltimore, identify the set of stars that never set
- These stars comprise the Circumpolar Region of our Local Horizon
- How does this region change with latitude?
- With Local Meridian on over Equatorial Grid: Define Transits & Sidereal Time
- Look up current Sidereal Time: https://www.localsiderealtime.com/
Written by Roy Prouty 20240318
Reviewed by